Optipedia • SPIE Press books opened for your reference.
Maxwell's Equations
Excerpt from Field Guide to Spectroscopy
Maxwell's equations of electromagnetism
Maxwell's equations are a set of four equations that describe the behavior of electric and magnetic fields and how they relate to each other. Ultimately they demonstrate that electric and magnetic fields are two manifestations of the same phenomenon.
In a vacuum with no charge or current, Maxwell's equations are, in differential form:
where E and B are the electric field and magnetic flux density, and ∇· and ∇× are the divergence and curl operators, respectively. The variables µ0 and ε0 are the fundamental universal constants called the permeability of free space and the permittivity of free space, respectively. In a vacuum with no electrical charges present, the mathematical solutions to these differential equations are sinusoidal plane waves, with the electric field and magnetic fields perpendicular to each other and to the direction of travel, having a velocity
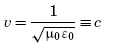
where c is recognized as the speed of light.
Maxwell's equations are macroscopic expressions; they apply to the average fields and do not include quantum effects.
Maxwell's Equations: General Form
In their most general form, Maxwell's equations can be written as
In the first equation, ρ is the free electric charge density. In the last equation, J is the free current density.
For linear materials, the relationships between E, D, B, and H are
Here, ε is the electrical permittivity, and µ is the magnetic permeability. For nonlinear materials, e and µ are dependent on the field strength. In isotropic media, e and µ are independent of position. In nonisotropic media, e and µ can be described as 3×3 matrices that represent the different values of permittivity and permeability along the different spatial axes of the medium. In all media, e and µ also vary with the frequency of the radiation.
To be consistent with Maxwell's equations, the magnitudes of the electric and magnetic field vectors must satisfy the following relationship:

Thus, in electromagnetic radiation, the electric field vector has a much larger amplitude than the magnetic field vector.
D. W. Ball, Field Guide to Spectroscopy, SPIE Press, Bellingham, WA (2006).
View SPIE terms of use.

